 |
| Home > Geometry > Topology |
Möbius bands
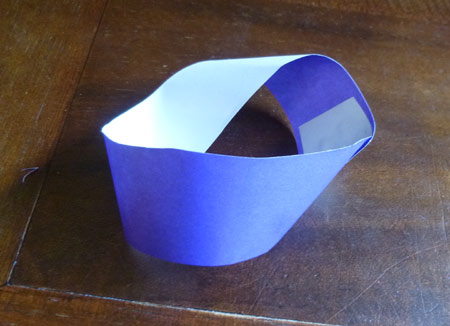 |
| A simple Möbius band (1, 1, B1) |
 |
Möbius band cut in half lengthways (½, 2, B2) |
 |
| Möbius band cut in thirds lengthways {1/3, 1, B1 + 1/3, 2, B2}
|
 |
| Möbius band with two twists
(1, 1, B2)
|
 |
| Möbius band with two twists cut in thirds lengthwise 3{1/3, 1, B2}
|
 |
| Möbius band with three twists
(1, 1, B3)
|
August Ferdinand Möbius was born on the 17th of November 1790. He made many discoveries, including the Möbius configuration of two tetrahedrons. Other discoveries include Möbius transformations, which involve complex numbers in projective geometry, and the Möbius function, an important function in number theory. However, his most famous discovery was of the Möbius band.
A story found in Fantasia Mathematica called "A. Botts and the Moebius Strip" refers to a man asking a lieutenant to paint a certain band. He asked for it painted on the outside but for not a drop of paint to be on the inside. Little did he know that the band was a Möbius band. It only has one face and one edge, as the half-twist connects what would be two distinct faces and edges. This is why, once the painting had finished, the inside had been painted completely. He then tried to wash it off but ended up washing the whole band clean.
I have also developed a notation for these bands. The band is a,b,Bc where a is the length (comparable to a certain band), b is the thickness, and c is the number of half-twists.
Call a normal cylindrical band 1, 1, B0. Cut it in half (always along its length) to get 2(1, 1/2, B0). This means that there are two separate B0s, each half the thickness. This, as most people would agree, is obvious. Cutting it in thirds gives 3(1, 1/3, B0). Again, this is obvious. It's far less obvious with the Möbius band 1, 1, B1. Cut it in half and you get a 2,1/2, B2. This is a longer but thinner band with a double twist. If you cut it in thirds you get a {2,1/3,B2 + 1, 1/3, B1}. Braces indicate that these bands are linked. Already it is far less intuitive than the B0. Things get even more complicated with B2. A1, 1, B2, when halved, gives 2{1,1/2,B2}. Unlike B0s, this gives linked bands. Similarly, when cut into thirds, it gives a 3{1, 1/3, B2}. This time these are all mutually linked.
All this is experiment. With B3 and above I have just tried to simulate them by drawing. A1, 1, B3, when halved, is conjectured to give a 2, 1/2, B6. However, this band is conjectured to be knotted. I still haven't developed notation for knotted bands or Borromean bands. Cutting and gluing paper seems too cumbersome to deal with knotted bands. Clearly a more high-tech tool is needed.
I have thought about a toy based on Möbius bands. There will be two main types of links: the flat link (just an unjoined band) and the twisted link (an unjoined band with a half-twist). These can be joined together to make Möbius bands, B2s, B3s, and so on. They will also be joined across their length. This could be taken apart to simulate cutting them in half.
Related entries
• Topology• Knot theory
• Flexagons